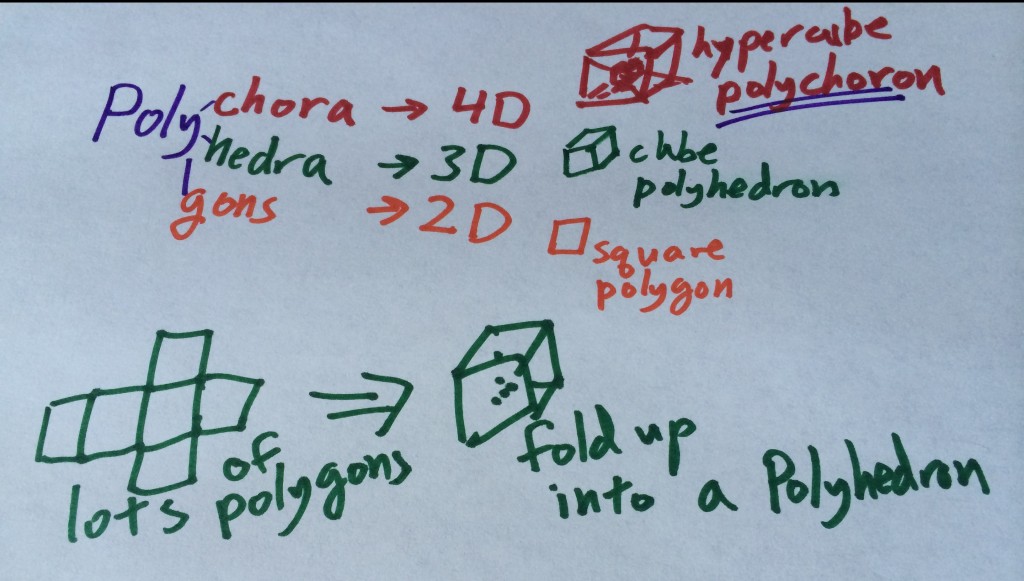
About two years ago, I decided to make a 120-cell out of hair bands. I had just come back from Gathering for Gardner 10, where I had helped my friend Zach Abel build a cool, giant rubber band sculpture. This sculpture was fantastic and I loved how boingy it was. I was incredibly inspired by his idea and technique of using elastic bands to create geometric sculpture, and I wanted to try making my own.
Shortly thereafter, Vi Hart asked me what my favorite polychoron was, a question to which I immediately answered “the 120-cell“.
See, my favorite number is five (a long-standing fact that first emerged when I was 5 years old), and, by extension, my favorite polygon is the five-sided pentagon. It naturally follows that my favorite polyhedron is the regular dodecahedron comprised of 12 regular pentagons. The 120-cell, made out of 120 regular dodecahedra, was basically a shoo-in for my favorite 4-polytope.
Thus, when I discovered CVS selling colorful hair bands by the hundred, it seemed obvious that I needed to make a giant, bouncy (projection of a) 120-cell out of them. Hair bands seemed like a natural thing to use for a 120-cell. Their natural stretch means that you don’t need to get the lengths exact (just close), they come in all kinds of colors, and they have a truly delightful sproing to them which encourages people to really interact with the final sculpture.
I immediately purchased 600 hair bands (buying out the CVS in question), and got started. I particularly like the way that the Schlegal diagram of the 120-cell shows all of the edges and vertices and has a regular dodecahedron in the very center and one on the outside, so I knew that my 120-cell was going to use that projection and have 1200 edges, so this seemed like a good start. I assumed that I would be able to return to the store once I’d used up my hair ties and buy some more. Unfortunately, what I hadn’t account for was that CVS doesn’t actually restock that quickly. Moreover, since only the innermost edges of the sculpture were a single band length, and I was using a color scheme to keep track of my place in the sculpture, I was going to need way more than 1200 hair ties.
In desperation, I tried hair tie shopping at a different local store, but they didn’t have the same hair bands. I ended up buying out 5 or 6 CVS all across the Bay Area to finally end up with enough hair bands for my sculpture.
With the structure completed, I ran into a different issue. The sculpture was going to be huge and was going to need to be stretched out from multiple corners. It was probably going to fill a whole room, and I didn’t actually have a spare room handy. Fortunately, my boyfriend had a small extra bedroom in his apartment, and, one weekend we took a bunch of 3M command strips and attached it above the bed, which was awesome, but also a bit strange. This picture was carefully cropped to hide the bed.
Not long thereafter we ended up taking the 120-cell down. Above one’s bed just isn’t a good permanent location, and the 120-cell languished in a bag for over a year before I had another opportunity to put it up in a small room in the CDG office.
Did I mention that the 120-cell is rather large?
Check out this Youtube video to learn more about the hair tie 120-cell.