math art – Andrea Hawksley

August 12, 2014August 17, 2014

6OSME

I spent the last week in Tokyo, Japan attending the 6th meeting on Origami, Science, Math, and Education (6OSME).

6OSME is an amazing gathering of researchers from all of the many disciplines relating to origami (computer science, mathematics, engineering, architecture, etc.), as well as serious origami artists. If you’ve ever doubted the validity of origami as a serious research discipline, then hearing a few talks here will surely change your mind. And if you think of origami as paper cranes made from a single folded square with no cuts, then the topics of these presentations might surprise you. There were way too many presentations for me to cover all of them, but here are a few of the topics that were covered to give you a sense of the breadth of origami research.

On the industrial engineering side, Gregory Epps kicked off the convention with a plenary talk on “Industrial Robotic Origami”, or the production of curved, folded metal by robots. Robots folding metal. ’nuff said.

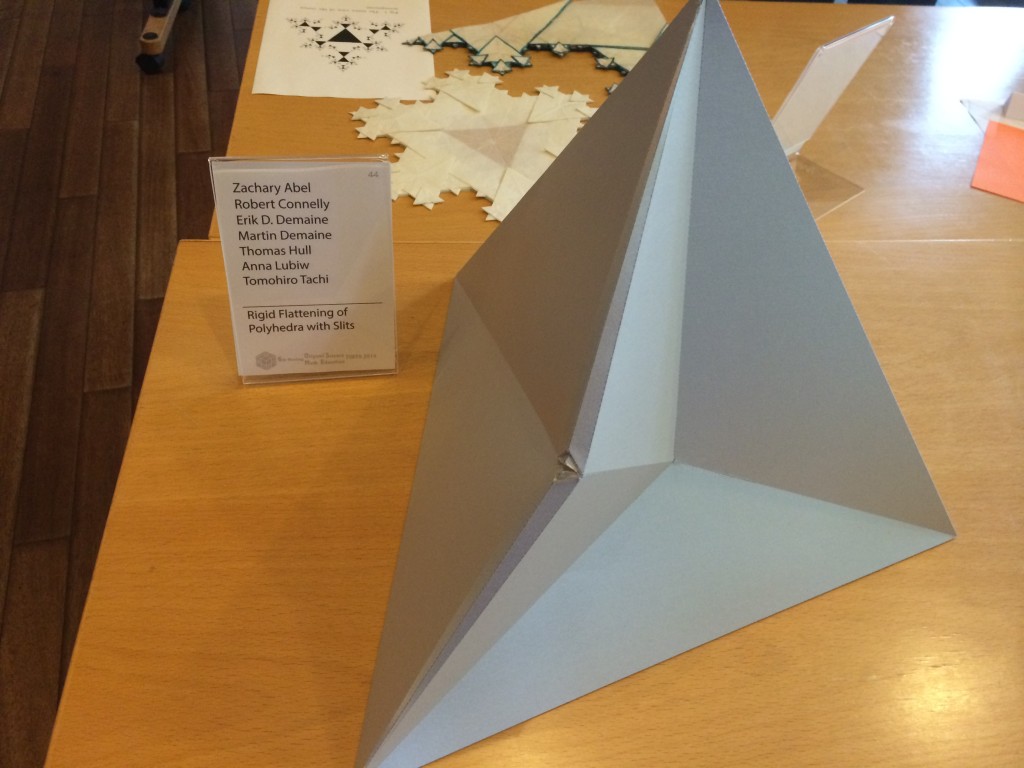
Mathematicians like to imagine that the world is made up of “ideal” paper, which is entirely rigid, bends only at fold lines and has no stretch. It’s also infinitely thin, of course. In this theoretical world, it turns out that you cannot squash paper polyhedra flat. You might have trouble achieving this world in practice, though – most paper is actually a bit stretchy. Abel, Connelly, Demaine, Demaine, Hull, Lubiw, and Tachi showed that by adding small slits it is possible to fold polyhedra flat in their work on “Rigid Flattening of Polyhedra with Slits”.

Most people don’t think of origami as involving cuts, but 6OSME actually had an entire session devoted to “pop-up” techniques. Yoshinobu Miyamoto showed off some impressively tall and sturdy structures made from single sheets in his presentation on “Rotational erection system (RES): origami extended with cuts”. The tallest one that he demonstrated looked to be about 4 feet tall, and it seemed clear that they could get way bigger with the correct materials.

Chris Itoh managed to pull the biological sciences into the conference with his talk on “The elusive technique of folding anatomical subjects”.

This talk on “Curved-folding convex polyhedra through smoothing” was from a highly architectural point of view. Suryansh Chandra, Shajay Bhooshan, and Mustafa El Sayed work in the research branch of an architectural firm, where they developed this technique so as to be able to create cool, “real-world” structures.

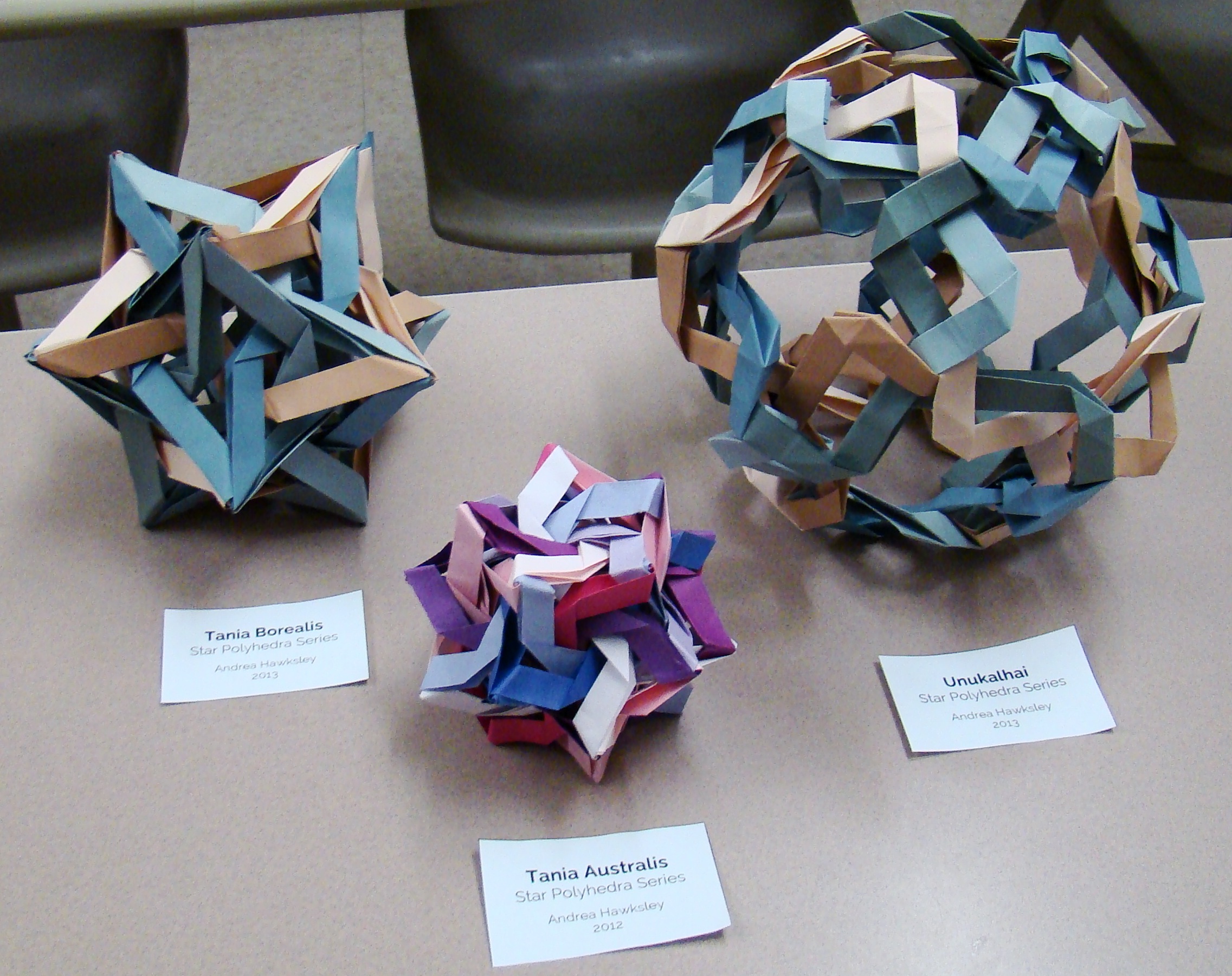
My own work was presented in the “modulars” session on origami made from multiple sheets of paper. I spoke on my star polyhedra series and the design process that I use to create these highly mathematical models.

The variety of origami sciences represented at OSME is amazingly impressive, and I don’t have the space to do it justice. I am missing a bunch of fantastic talks just because I was focusing more on breadth than on depth, but I haven’t even covered the full breadth here. You’ll have to check out the full papers (when they come out) to get the scoop.

OSME is held only once every four years, so if you want to attend the next one you will have to wait for a while. On the other hand, you’ll have plenty of time to prepare your presentation!

June 4, 2014June 12, 2014

Fibonacci Lemonade

How would one make mathematical cuisine? Not just food that looks mathematical (like math cookies), but something that you truly have to eat and taste in order to experience its mathematical nature.

Henry Segerman proposed this question, and today we had our first experience tasting the answer. A masterpiece of mathematical art, the answer I came up with is simple enough that anyone can make it at home, surprisingly visually beautiful, delicious…

Layered Drinks!

Not just any layered drinks, of course. In our layered lemonade, the intensity of flavors as you go down the layers increases exponentially. The sugar and lemon juice proportions for the same quantity of liquid increase according to the Fibonacci sequence. The sweeter layers are denser and naturally keep separated lower down. Indeed, the nature of layered drinks is that they are monotonically increasing in sweetness (and/or decreasing in alcohol). Thus, there is an intrinsic mathematical property to all layered drinks.

Additionally, the ratio of sugar to lemon juice in our lemonade isn’t constant. The top layer of our drink is 1 part lemon, while the second layer is 1 part sugar syrup. Following the Fibonacci rule, each subsequent layer has proportions that are the sum of the previous two layers proportions.

Layer 3 is 1 part lemon, 1 part sugar. Layer 4 is 1 part lemon, 2 parts sugar, and so on.

Generically, for layer n > 2, there are fib(n-1) parts sugar and fib(n-2) parts lemon juice. These are adjacent Fibonacci numbers, so as the drink is consumed the ratio of lemon to sugar approximates the Golden Ratio. This drink may be the worlds first tastable example of the relationship between the Fibonacci sequence and the golden ratio!

Surprisingly, using golden ratio relative proportions of lemon juice and simple syrup actually seems to make rather good lemonade. When consumed, the beverage starts out fairly flavorless, then rapidly ramps up. It also alternates between being a bit sweet to being slightly sour (of course this is a matter of personal taste), as the approximation of the golden ratio alternates between being slightly high and slightly low.

You too can make Fibonacci lemonade and experience the taste of exponential flavor, the golden ratio, and the Fibonacci sequence. Just follow the recipe below!

Ingredients and Materials:

Lemon Juice
SImple Syrup (1 cup sugar dissolved in 1 cup water)
Water
Food Coloring (optional but makes it pretty)
Ice or a spoon for slow pouring (the ice free strategy is much harder)

The Method

You must start with the sweetest and densest layer and work your way backwards up the drink. I describe the layers for a 7 layer drink below, although you may choose to make a different number of layers to start. The drink could also be made without the first layer, in which case it is neatly just two offset increasing Fibonacci sequences, one per ingredient.

First, fill your glasses with ice. Then, do the following steps for each layer. Finally, sip your mathematical masterpiece.

Add the proportions of lemon juice and simple syrup indicated below to your liquid measuring cup.
Add food coloring if desired.
Fill measuring cup to the 4 oz. (1/2 cup) line.
Stir to blend all ingredients in your measuring cup.
Slowly pour a layer from your measuring cup into your drink glasses. You want to pour directly onto an ice cube, the ice cubes are there to slow down your liquid as it goes down the cup and to help keep the layers distinct. (You can pour the first layer normally)

The Layers

1 tsp. lemon juice
1 tsp. simple syrup
1 tsp. lemon juice, 1 tsp. simple syrup
1 tsp. lemon juice, 2 tsp. simple syrup
2 tsp. lemon juice, 3 tsp. simple syrup
3 tsp. lemon juice, 5 tsp. simple syrup
5 tsp. lemon juice, 8 tsp. simple syrup

Tips

Many simple syrups are 2 parts sugar to 1 part water. If yours is like this, halve the amount of sugar you are using (or it will probably be far too sweet).
For a more authentic, less watered down experience, you need to make your drinks without ice. This is much harder, and I don’t actually recommend it unless you are patient or really know what you are doing. You can find directions for layering without ice here.

June 4, 2014June 12, 2014

Polly wants a Parrotohedron

Last week, I looked out the window of my office and saw a parrot. Two of them actually. Apparently San Francisco is home to a wild colony of escaped (released?) Cherry Headed Conures. There is even a documentary about them, but somehow I had seen nor heard of them before.

Walking back to my desk, I had a moment of inspiration.

Parrot. Pyrite. Pyritohedron.

I needed to make a Parrotohedron.

The internet is kind of amazing, and I quickly found a website that would sell me parrots in 3 different colors for 39 cents apiece. I think they are supposed to be used as accents for floral centerpieces; I have no idea how anyone is making a profit selling them at that price.

A parrotohedron obviously needs to be pyritohedral, so I started by arranging six sticks pyritohedrally for my parrots to perch on. Then, I arranged 12 parrots (one per stick end) such that each parrot had a friend (and represented one face of a pyritohedron).
Squawk! It’s a Polly-hedron.

But will there be a pirate-ohedron? Only time will tell.

ps. Arrr. Where be my two-dimensional parrot?
pps. Polly gone.

May 22, 2014June 12, 2014

‘Topological’ Origami, the Star Polyhedra Series

I first posted about an origami model based on a Star Polyhedron in November 2012, but I knew at the time that many more models were possible based off of a similar idea of taking a Star Polyhedron and removing parts of each face such that some underlying topology of the polyhedra was maintained, but the faces no longer intersect. These representation of a star polyhedron can be thought of as ‘topological’ as they emphasize the internal connectedness of these self-intersecting figures.

Since then, I have folded some more polyhedra following the same idea, and started naming them after actual stars (seeing as they are “star” polyhedra). I will presenting on some of the math and design thoughts for this series at the 6th International Meeting on Origami in Science, Mathematics and Education (6OSME) in Tokyo and displaying two of them at Bridges 2014 in Seoul, Korea later this summer.

Unukalhai, below, is a ‘topological’ model based on the Small Triambic Icosahedron and is composed of 60 rectangular sheets of paper folded into identical origami units. Each face is represented by a 3-pronged spiral, and can be thought of as a subsection of a small triambic icosahedron, chosen such that the model can be joined together without self-intersection. Unukalhai was made out of five colors of paper to highlight the natural five-coloring of the icosahedron.

Tania Australis was the first star polyhedra origami piece that I folded, and has been mentioned on this blog before. It is composed of 30 identical ‘S’ shaped pieces that each represent one face of a Great Rhombic Triacontahedron and was inspired by George Hart’s Frabjous sculpture.

Tania Borealis is composed of 30 ‘S’ shaped pieces, put together in the shape of a Medial Rhombic Triacontahedron. It complements Tania Australis, but is much more fragile, so I do not plan to put it on display in Asia.

April 17, 2014June 12, 2014

Six Card Ball

My gift for Gathering For Gardner 11 was a set of pre-cut playing cards that can be used to assemble a cute geometric construction. The final construction looks like this:

It’s a fun design where the interior figure is clearly a dodecahedron, but, since each card represents two faces of the dodecahedron, the symmetry group represented is pyritohedral.

Here is a template that you can print out and cut up to make your own set of cards for this construction.

Your slotted cards should look like this:

You will need six of them to create your ball. Each card should be folded in half “hamburger-style”.

You can join two cards, slotting the two short cuts on one side of one card into two adjacent long cuts on another. All the cards slot into all of the other cards in this same way.

Adding a third card starts to get tricky. The three cards slot together to form a three way join. This is pretty difficult to explain, but here are a bunch of pictures. Don’t worry! Once you have one three-way corner done, the rest are put together the same. By the time you finish, you’ll be a pro!

Now is a great time to take a peek at the inside of your ball before you start closing it up. Can you see the dodecahedron forming?

The rest of this model pretty much follows from the steps previously described. Keep adding pieces like above until your ball is complete! Here are some pictures of the finished ball from different angles.